Difference between revisions of "Huygen's pendulum"
| Line 40: | Line 40: | ||
Also describe how it has been integrated into collections, their role in each storytelling (if part of an argumental story)... | Also describe how it has been integrated into collections, their role in each storytelling (if part of an argumental story)... | ||
--> | --> | ||
| − | The exhibit is part of a | + | The exhibit is part of a series on the cycloid and its properties, included in the exhibition “Beyond the compass” from Il Giardino di Archimede. The other exhibits are: |
| − | + | ||
| + | # Construction of a cycloid by rotating a wheel on a whiteboard, | ||
| + | # Brachistochrone / isochrone property: by leaving a ball fall through a different tracks, we see that the cycloid minimizes falling time, and time is independent of the starting point. | ||
| + | # Evolute property by a wooden profile on a whiteboard with a string. | ||
==Resources== | ==Resources== | ||
Latest revision as of 22:46, 26 August 2021
| Huygen's pendulum | |
|---|---|

| |
| On display at | Il Giardino di Archimede |
| Type | Hands-on |
| Topics | geometry, cycloid, history |
This exhibit is part of the exhibition Beyond the compass at Il Giardino di Archimede (Florence, Italy).
Description
In the exhibit, two pendulums are compared. The first one (left) is a free pendulum, moving along a circumference. The second one (right) is a Huygen’s, or cycloidal pendulum, where the weight moves along a cycloid. Two photocells measure their periods, which are visualized on a screen.
The two pendulums are as long as the ceiling allows, have the same length, and are attached to a wooden structure that supports the contraption. Each pendulum has a double string, making a V shape, so the pendulum has two anchor points and oscillates in only one plane (as opposed to a single string pendulum that would move in all directions).
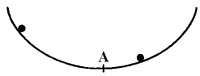
To make Huygen’s pendulum, the string is attached on the top to a wooden curved template on each side. When the pendulum oscillates, the string is forced to rest in contact witfh the template, adopting the shape of a cycloid arch.
A sensor flips its state each time that the weight of the pendulum crosses the lowest point of its trajectory. The computer measures the state change and displays on the screen the duration of the oscillation period. The screen has two graphs, one per pendulum.
Activities
As the pendulums start oscillating, we can see that while the period of the ordinary pendulum decreases with the amplitude of its oscillations, the one of the cycloidal pendulum is strictly constant. The screen is non-touch, the interface allows to record, pause and reset the graphs, although that would even be unnecessary by having the graph reset itself after a few seconds. The main interaction of the visitor is not by touching the screen, but by releasing gently the weight of each pendulum at different heights. In Giardino, this exhibit comes last after other exhibits introducing the cycloid’s main properties (see Museology below). This exhibit helps to visualize an application of the cycloid through the Huygens’ pendulum and to understand better its mathematical aspects, completing the exposition on the cycloid's properties.
Mathematical background
Galileo had observed how the oscillations of a pendulum take more or less the same time, independently from the amplitude. Pendulum clocks use this principle but, in reality, the pendulum’s oscillations are not exactly isochronous: the time it takes to complete an oscillation does depend on the oscillation’s amplitude, and it is longer for wider oscillations. Only for very small oscillations (as in clocks) we can consider the time substantially constant.
How must a pendulum be so that all its oscillations require strictly the same time, in Greek iso-chronous? More precisely, along which type of curve must a body oscillate so that the oscillations are perfectly isochronous? The answer is the cycloid.
But, how to make a pendulum oscillate along a cycloid instead of a circle? The trick is to use some profile so that the string rests in contact to it and describes what is called mathematically the evolute of the profile curve. It is a remarkable fact that the evolute of a cycloid is another cycloid equal to the first (this can be shown in another module). This is how the isochronous Huygen’s pendulum is constructed.
History and museology
The exhibit is part of a series on the cycloid and its properties, included in the exhibition “Beyond the compass” from Il Giardino di Archimede. The other exhibits are:
- Construction of a cycloid by rotating a wheel on a whiteboard,
- Brachistochrone / isochrone property: by leaving a ball fall through a different tracks, we see that the cycloid minimizes falling time, and time is independent of the starting point.
- Evolute property by a wooden profile on a whiteboard with a string.