Pythagoras' theorem exhibits
| Pythagoras' theorem | |
|---|---|
| |
| On display at | Many math museums |
| Type | Hands-on |
| Topics | Pythagorean theorem |
On this page, we include many modules around the Pythagorean theorem.
Contents
Description
Those exhibits are intended to display and verify the Pythagoras' theorem with a particular setting. Here a few of the contraptions used in museums:
Pythagorean liquid disk
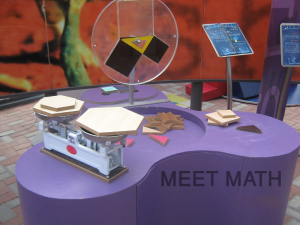
An acrylic disk that can be rotated on support. In its interior, a right triangle and three squares over its sides. The squares are empty, and a liquid can flow between the three squares. By rotating the disk, the liquid that fills the two smaller squares can be made to fill the bigger one.
Decomposition puzzles
Those puzzles are usually made of wooden pieces. A right triangle is glued on the table. With the wooden pieces, two squares can be constructed over the legs of the triangle, or re-arranged to form a single square over the hypothenuse. Sometimes the shapes to construct over the sides are not squares, but other shapes polygons, giving an additional nice touch.
Pythagorean balance
Several pieces of different shapes (hexagons, half-circles...) in sets of three, all the three in each set with the same shape but different size, that fit the size of the sides of a fixed right triangle. A balance (usually of type Roberval) allows comparing weights, to verify that no matter the shape, the sum of the weights of the two smaller is equal to the weight of the bigger.
Other jigsaw constructions
We include here the Pythagorean window, the Pythagorean rings, lunulae constructions, and many others that can be found in museums.
Pythagorean "liquid" disk, Pythagorean balance, and Pythagorean decompositions at Meet Math Museum
Pythagorean balance at Experiminta.
Pythagorean decomposition at Il Giardino di Archimede.
Pythagorean lunula at Il Giardino di Archimede.
Pythagorean decomposition at Il Giardino di Archimede.
Pythagorean sculpture at Il Giardino di Archimede.
Hinged Pythagorean decomposition at Mathematikum.
Pythagorean decomposition of a (3,4,5) right triangle with area units, at Mathematikum.
Pythagorean balance at Mathematikum.
Pythagorean balance at MMACA.
Pythagorean window at MMACA.
Pythagorean rings at MMACA.
Pythagorean paradox at MMACA.
Activities and user interaction
This super-classical subject is still a big success in math museums. This is due to the familiarity of the public with the theorem (but not with these physical realizations), the fact that it is closely related to the school curriculum (teachers demand it), the feeling of understanding, and for the adult public the pleasure of being surprised by something supposedly so exhausted. Few instructions are necessary to induce users to handle the exhibits. However, it is important to make some remarks (by panels or by mediators):
- Underline the relationship between weight and areas (due to the fact that all shapes have the same thickness).
- That something that looks true is not always true. Compare with some apparent paradoxes where some misalignments induce to see vanishing areas.
- Most importantly, remark that a verification is not a proof. The proof must be an argument. In particular, why does that particular fact remains true for any other right triangle? Why the argument does not depend on this physical realization?
Mathematical background
There are hundreds of known proofs of the Pythagorean theorem. Many of them are susceptible to be adapted to a museum exhibit.
History and museology
Almost every math museum has some exhibit related to the Pythagorean theorem, some of the reasons are pointed above. Some exhibits, like the liquid disk or the Pythagorean balance, are widely spread. Others are much less known and still surprise the most experienced visitors. We are aware of those of Mathematikum; MMACA, which makes special teacher training around that topic; and Il Giardino di Archimede, that created an exhibition named “Phythagoras and his theorem”.












